Knitting Batik

在 $n\times m$ 的矩形中给每个格子任意涂上 $k$ 中颜色，同时给出两个大小相同的子矩形位置及其大小，求有多少种涂色方案使两个子矩形涂色完全相同。

Mr. Chanek wants to knit a batik, a traditional cloth from Indonesia. The cloth forms a grid $ a $ with size $ n \times m $ . There are $ k $ colors, and each cell in the grid can be one of the $ k $ colors. Define a sub-rectangle as an ordered pair of two cells $ ((x_1, y_1), (x_2, y_2)) $ , denoting the top-left cell and bottom-right cell (inclusively) of a sub-rectangle in $ a $ . Two sub-rectangles $ ((x_1, y_1), (x_2, y_2)) $ and $ ((x_3, y_3), (x_4, y_4)) $ have the same pattern if and only if the following holds: - they have the same width ( $ x_2 - x_1 = x_4 - x_3 $ ); - they have the same height ( $ y_2 - y_1 = y_4 - y_3 $ ); - for every pair $ (i, j) $ where $ 0 \leq i \leq x_2 - x_1 $ and $ 0 \leq j \leq y_2 - y_1 $ , the color of cells $ (x_1 + i, y_1 + j) $ and $ (x_3 + i, y_3 + j) $ are equal. Count the number of possible batik color combinations, such that the subrectangles $ ((a_x, a_y),(a_x + r - 1, a_y + c - 1)) $ and $ ((b_x, b_y),(b_x + r - 1, b_y + c - 1)) $ have the same pattern. Output the answer modulo $ 10^9 + 7 $ .

The first line contains five integers $ n $ , $ m $ , $ k $ , $ r $ , and $ c $ ( $ 1 \leq n, m \leq 10^9 $ , $ 1 \leq k \leq 10^9 $ , $ 1 \leq r \leq \min(10^6, n) $ , $ 1 \leq c \leq \min(10^6, m) $ ) — the size of the batik, the number of colors, and size of the sub-rectangle. The second line contains four integers $ a_x $ , $ a_y $ , $ b_x $ , and $ b_y $ ( $ 1 \leq a_x, b_x \leq n $ , $ 1 \leq a_y, b_y \leq m $ ) — the top-left corners of the first and second sub-rectangle. Both of the sub-rectangles given are inside the grid ( $ 1 \leq a_x + r - 1 $ , $ b_x + r - 1 \leq n $ , $ 1 \leq a_y + c - 1 $ , $ b_y + c - 1 \leq m $ ).

Output an integer denoting the number of possible batik color combinations modulo $ 10^9 + 7 $ .

3 3 2 2 2
1 1 2 2

32

4 5 170845 2 2
1 4 3 1

756680455

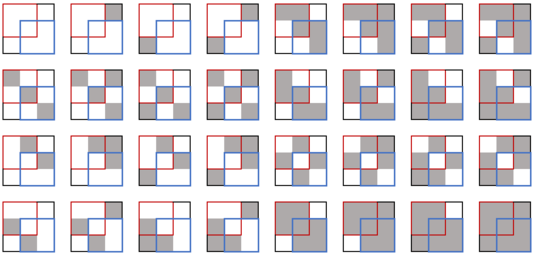
The following are all $ 32 $ possible color combinations in the first example.